Special Relativity in 14 Easy (Hyper)steps
9. Quantitative description of nonsimultaneity
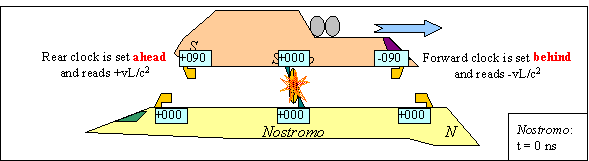
Let's assume that all clocks on a ship are synchronized in
the ship's rest frame, and that the clocks on each ship mounted
next to the (smashed) antennas happened to read zero when the collision
occurred. Let's also assume that each ship has a clock installed at each
periscope.
Here's what we know:
-
According to observers on each ship, their own clocks work
perfectly well, and are spaced apart by 150 feet.
-
According to observers on each ship, clocks on the other
(moving) ship run too slowly, by a factor
of
.
For every 25 nsec which passes in a ship's rest frame, only 20 nsec will
pass on the moving ship's clocks. In addition, the distances between adjacent
clocks on the other (moving) ship are Lorentz-contracted to 120 feet.
-
All clocks mounted by periscopes will happen to read 150
nsec when light arrives at the corresponding periscope. (See the previous
slide.)
-
All observers will see the other (moving) ship traveling
at 0.6 c, and the light from the explosion traveling at c.
Here's an animated GIF of the collision from Nostromo's
perspective,
using what we know.
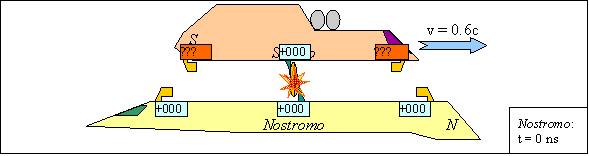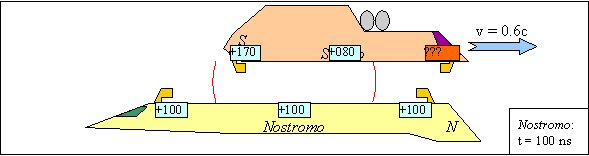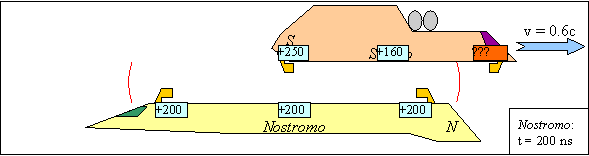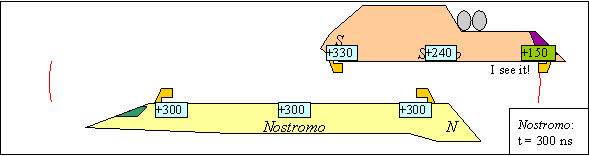
We want to calculate how much out-of-synch the clocks
on the moving ships are. Do it like this for the forward (moving) clock:
-
Figure out how long it takes light to reach the forward periscope
on the moving ship, according to clocks on the
stationary ship. If the light reaches the periscope Dt
after the explosion, we'll have
cDt = vDt
+ L
where L is the distance from the antenna to a periscope
in
the rest frame of the moving ship. (L = 150 feet; the equation
just says that the distance traveled by the light is the same as the sum
of the distance traveled by the moving periscope [while the light was en
route] and the Lorentz-contracted distance from the antenna to the periscope.)
-
Figure out how much time passed on the moving
ship's clocks during the time interval Dt
which was measured by the stationary ship. (This time interval began with
the destruction of the antennas and ended when observers on the stationary
ship saw light reaching the forward periscope on the moving ship.) This
one's easy: clocks on the moving ship tick slowly, so the amount of time
passing on them is
Dt´ =
Dt.
We know the forward clock on the moving ship read 150
nsec when the light from the explosion arrived (this is just L/c, where
L is the moving ship's rest length).
As seen from the stationary ship, the
moving ship's forward clock must have read 150 - Dt´
when the explosion first took place.
When you combine all the algebraic terms, you'll find that
the forward clock reads an earlier time than the center clock, as you can
see in the animation. The result is
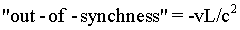
Clocks towards the front of the moving ship read earlier
times than clocks towards the back of the moving ship. At the time of the
collision, according to observers on Nostromo, things looked like
this:
