(where the choice of units may require an additional multiplicative constant
to be included in the force law)
Here's the usual way in which magnetic fields and
forces are presented:
(where the choice of units may require an additional multiplicative constant
to be included in the force law)
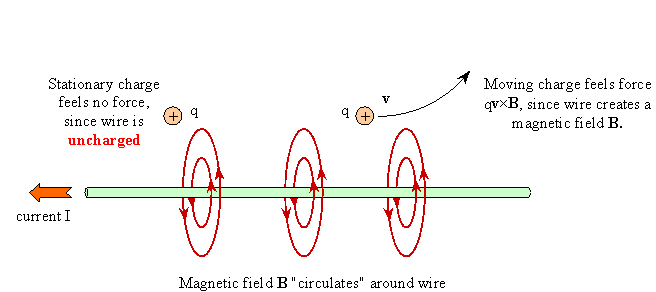
A diagram: notice that the moving charge experiences
a force directed away from the wire, just as if it were being repelled
by a wire carrying a net positive charge.
(However, since the stationary charge feels no force, we conclude that
the stationary wire is electrically neutral.)
Let's take a micro-look inside the wire. Current flowing to the left corresponds to electrons flowing to the right with, say, speed u. Keep in mind that the wire has as many positive metal ions as negative electrons per meter (since it is uncharged).
The first diagram is from the perspective of observers at rest with respect to the wire, the second from observers who are moving to the right with the same speed as the electrons.
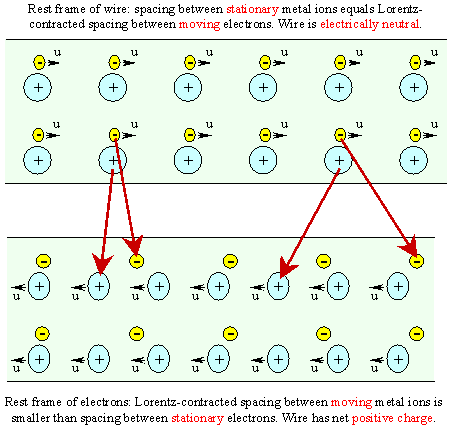
An observer moving to the right will see the wire as having net positive charge since the electron spacing "de-contracts" while the metal ion spacing decreases: there are fewer electrons, but more metal ions per meter of wire. (This is also true for observers who are not moving at the same speed as the electrons: there's more Lorentz (de)contraction of one sign of charge than the other.)
From the perspective of an observer moving to the right
(holding the test charge in the first figure),
the wire will be moving to the left, and will exert an
upwards force on the test charge since the wire carries a net positive
charge. To this observer, it's not a magnetic force at all, but a Coulomb
force due to the presence of an electric field coming from the charged
wire.
This is why there are magnetic fields: their effects are
a manifestation of the fact that charges exert electrostatic forces on
other charges, and that special relativity makes current-carrying wires
which are neutral in one frame appear charged in another frame.
