The calculations required in the following problem aren't difficult . What IS hard is to figure out how to think about the system in order to find the easy way to solve the problem. If you're able to get it, come see me. We'll discuss your solution and, if it's right, I'll write you a letter of recommendation when you need one for grad school, a job, whatever. Good luck!
The equivalence principal postulates that physics in a uniform gravitational field is, locally and for small particle speeds, the same as physics in an accelerated frame of reference. A number of consequences of General Relativity can be derived directly from the equivalence principal combined with Special Relativity. One of these is the fact that synchronized clocks at rest in a gravitational field lose their synchronization if their separation isn't perpendicular to the gravitational field.
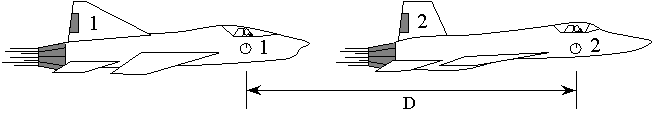
Imagine that a pair of powerful aircraft with synchronized clocks are separated by a distance D along the x axis in their rest frame. When each plane's clock reads zero, it accelerates with constant acceleration a (as measured by the pilot, in the instantaneous rest frame of the airplane) in the x direction until an amount of time T has passed on the aircraft's clock. At that time the aircraft resumes flying with constant velocity. The planes' speeds are always small compared to the speed of light.

The Lorentz transformations are
b describes the motion of the primed coordinate axes relative to the unprimed frame.
You may find it easiest to think of the acceleration as consisting of a series of discrete jumps during which a plane's velocity changes instantaneously by a finite (but small) amount. As a result, you will be analyzing motion in which an airplane keeps shifting from one intertial frame to the next frame until the clock it carries reads T.
Calculate the (very small) difference in the readings of the two clocks after the planes have finished accelerating, as seen by observers in the final rest frame of the aircraft. Evaluate your answer for the specific case a = 10 m/sec/sec , T = 100 seconds, D = 1000 meters. You may produce an answer which is correct to lowest order in b.