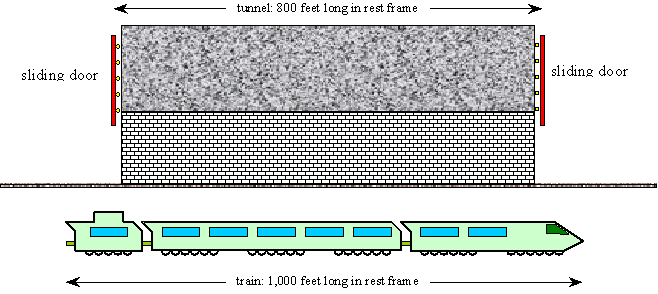
Here's how this one works. We have a tunnel, 800 feet long in its rest frame, with doors on each end which can be used to seal the tunnel. The train is 1,000 feet long in its own rest frame, as shown in the illustration:

Tunnel rest frame:
The train travels at speed 0.8 c so that its Lorentz-contracted length allows it to fit entirely inside the tunnel. When (synchronized in their rest frame) tunnel clocks by both doors read zero, just as the train is neatly centered inside the tunnel, the doors slam shut, trapping the entire train inside the tunnel. The front of the train crashes through the right-side tunnel door 125 nanoseconds later, but the (closed) left-side door was able to close without interfering with the train.

Train rest frame:
From the train's rest frame, things look rather different: the Lorentz-contracted tunnel rushes towards it as shown in the following figure.
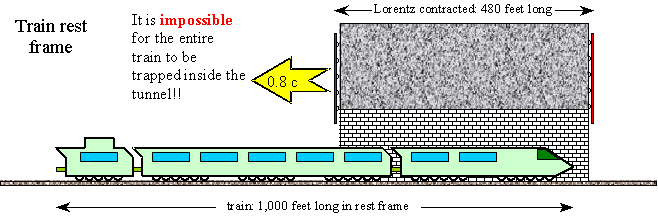
The tunnel is much too short
to be able to trap the train entirely inside itself, yet both doors will
drop, and neither will touch the train until the front of the train strikes
the already-closed right-side door.
How can this be possible?